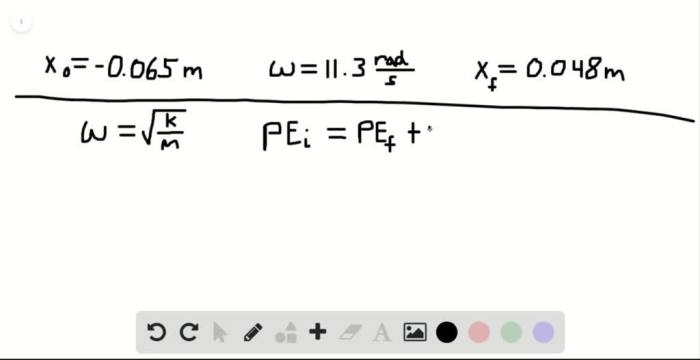
When a horizontal spring is lying on a frictionless surface, it presents a fascinating scenario where the fundamental properties of springs interact with the absence of frictional forces. This interplay gives rise to intriguing dynamics, oscillations, and energy conservation principles that are explored in this comprehensive analysis.
Delving into the characteristics of the spring, we examine the relationship between spring constant, displacement, and potential energy. The frictionless surface, a crucial aspect of this system, eliminates energy dissipation due to friction, allowing us to isolate and study the spring’s behavior.
Horizontal Spring
A horizontal spring is a flexible device that stores energy when stretched or compressed. It consists of a coiled or helical structure made of a springy material, such as steel or rubber, and is designed to exert a restoring force when displaced from its equilibrium position.The
fundamental properties of a horizontal spring include its spring constant, displacement, and potential energy. The spring constant (k) is a measure of the stiffness of the spring, which determines the amount of force required to stretch or compress it by a unit distance.
Displacement (x) represents the distance the spring is stretched or compressed from its equilibrium position. Potential energy (U) is the energy stored in the spring due to its deformation, and it is directly proportional to the square of the displacement.
Frictionless Surface
A frictionless surface is a hypothetical surface that offers no resistance to motion. In the context of spring dynamics, a frictionless surface is important because it eliminates the effects of friction, which can introduce damping and alter the behavior of the spring-mass system.
On a frictionless surface, an object attached to a spring can move freely without experiencing any frictional forces that would impede its motion.
Spring-Mass System
A horizontal spring-mass system consists of a spring attached to an object of mass (m). When the spring is stretched or compressed, it exerts a restoring force on the object, causing it to oscillate back and forth around its equilibrium position.
The system can be illustrated as follows:[Diagram of a horizontal spring-mass system]The components of the system include the spring, the mass, and the surface on which the system is placed. The spring provides the restoring force, the mass determines the inertia of the system, and the surface provides support for the motion.
Oscillations, A horizontal spring is lying on a frictionless surface
Oscillations in a horizontal spring-mass system refer to the periodic motion of the object attached to the spring. When the spring is displaced from its equilibrium position, the restoring force causes the object to accelerate back towards the equilibrium point.
However, as the object approaches the equilibrium position, its kinetic energy is converted into potential energy, causing it to slow down and eventually reverse direction. This process repeats itself, resulting in the object oscillating back and forth.The frequency of oscillations is determined by the spring constant and the mass of the object.
A stiffer spring (higher spring constant) will result in a higher frequency, while a heavier mass will result in a lower frequency. The amplitude of oscillations is determined by the initial displacement of the spring.
Answers to Common Questions: A Horizontal Spring Is Lying On A Frictionless Surface
What is the significance of a frictionless surface in this system?
A frictionless surface eliminates energy loss due to friction, allowing us to isolate and study the spring’s behavior without the confounding effects of frictional forces.
How does the spring constant affect the system’s oscillations?
The spring constant determines the stiffness of the spring. A higher spring constant leads to faster oscillations with a smaller amplitude, while a lower spring constant results in slower oscillations with a larger amplitude.
What is the role of damping in this system?
Damping introduces energy dissipation into the system, causing oscillations to gradually decay over time. Different types of damping, such as viscous damping and Coulomb damping, have distinct effects on the system’s behavior.